A Series of Snowflakes
Published on 6 December 2012
Subverted snowflakes or delicate doilies?

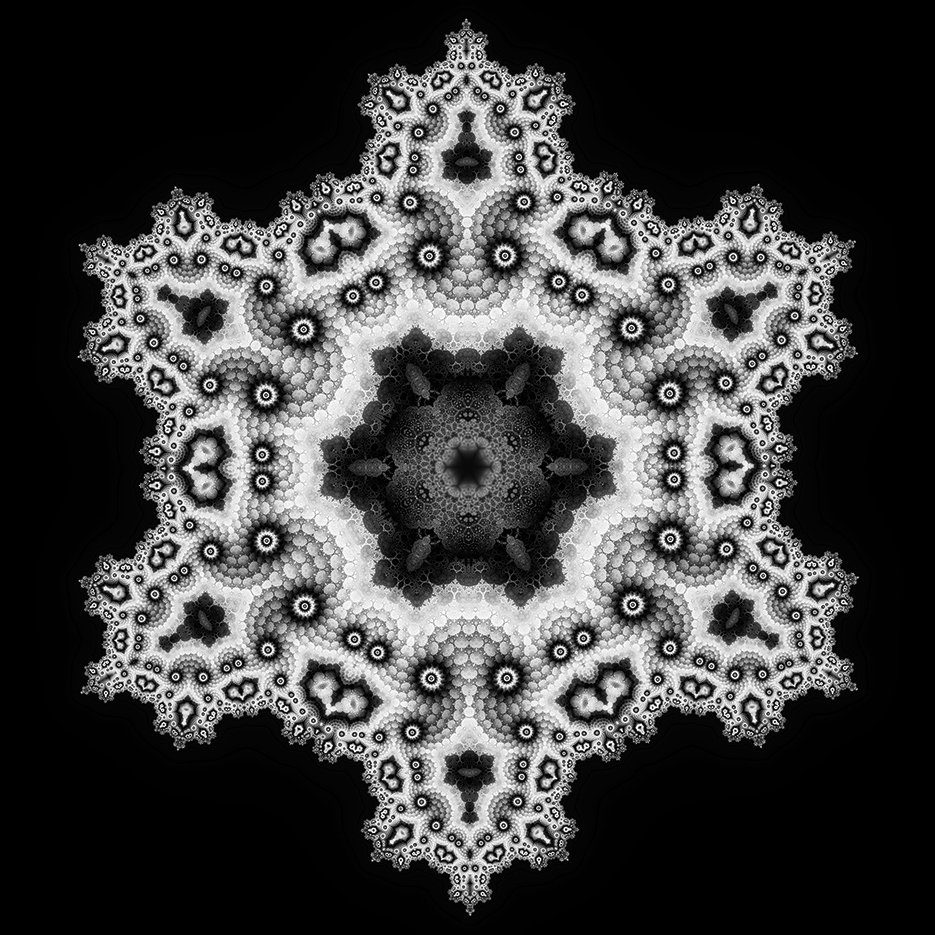
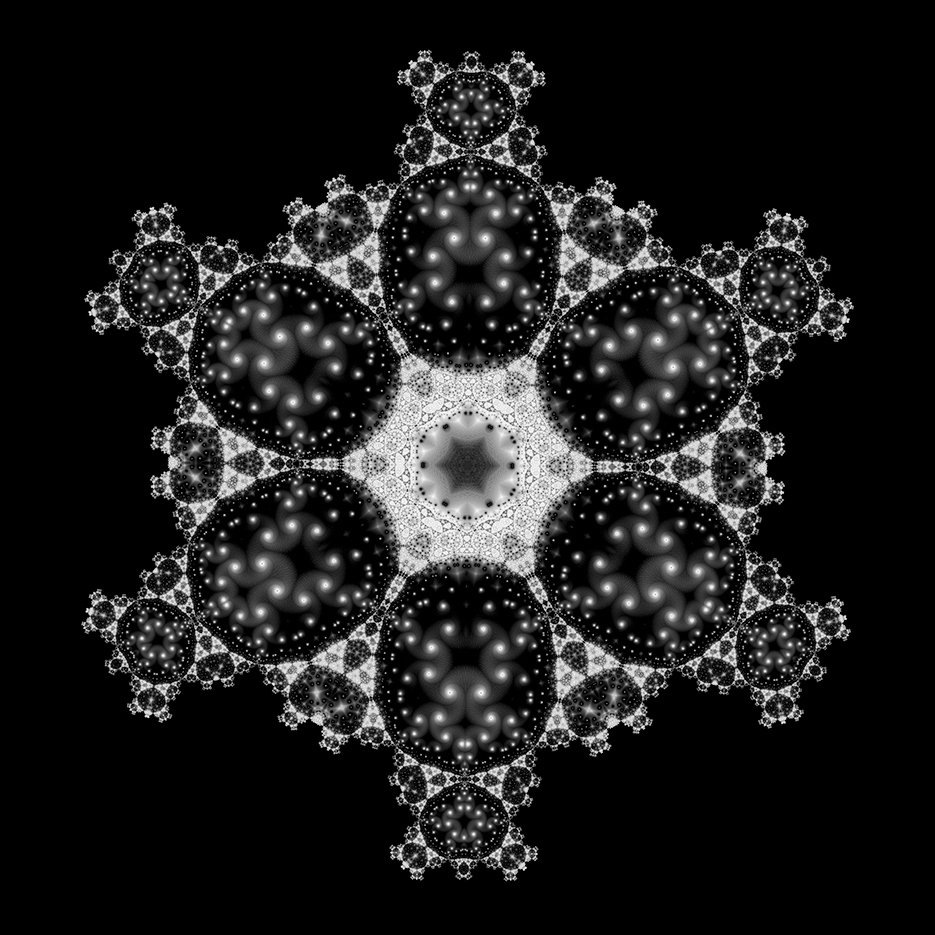
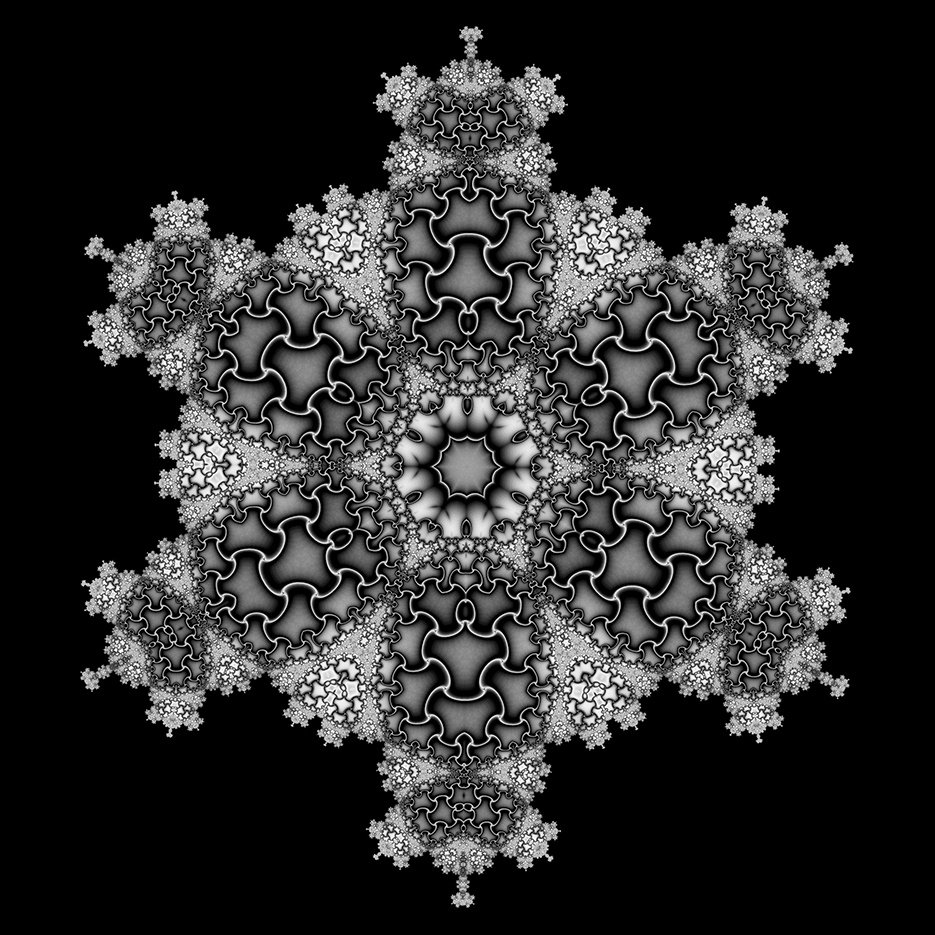
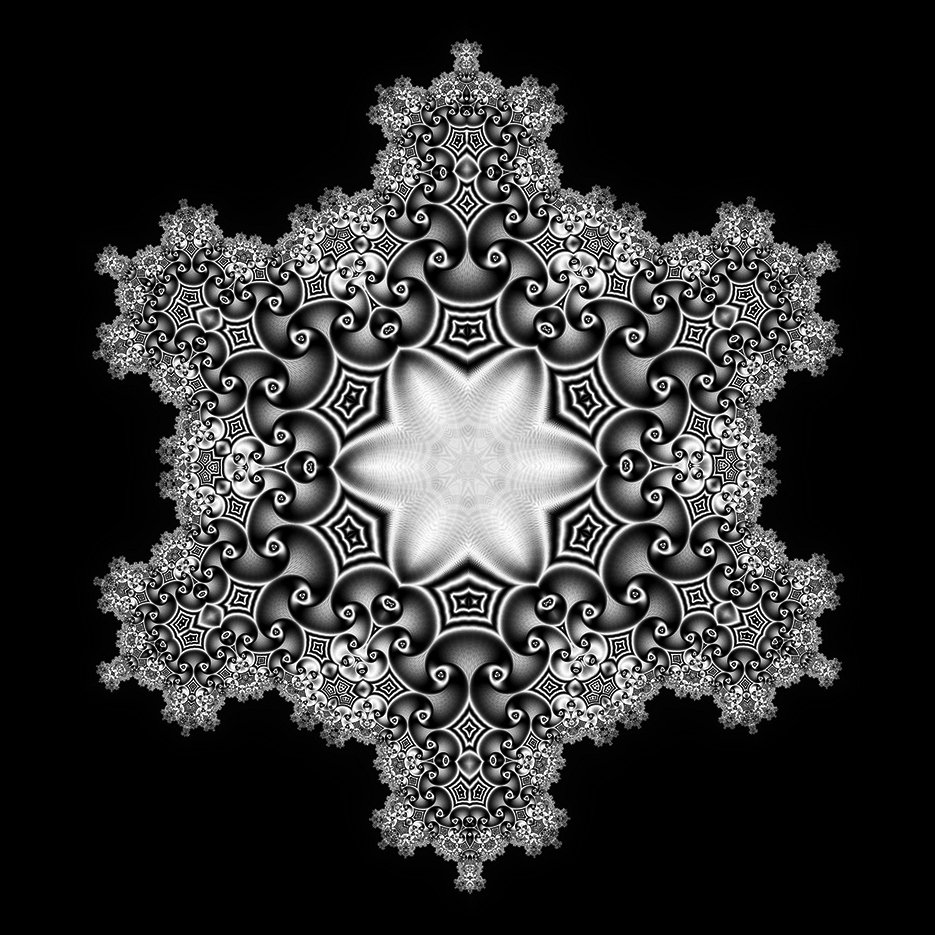
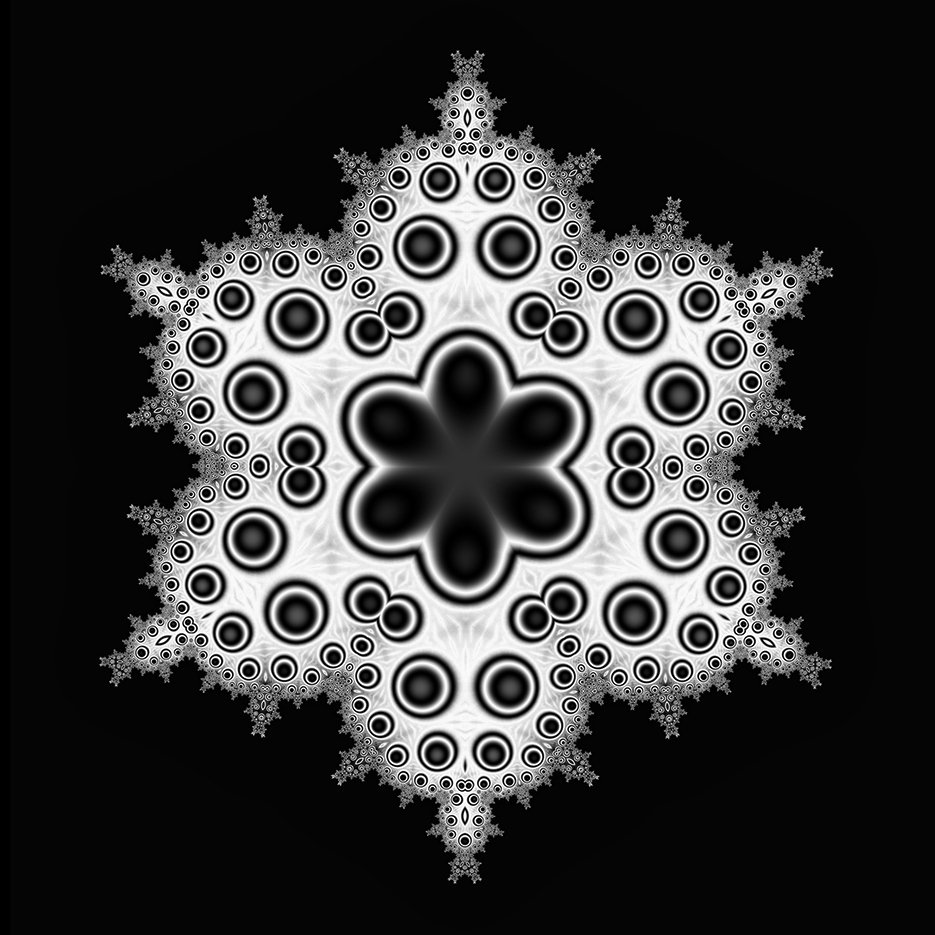
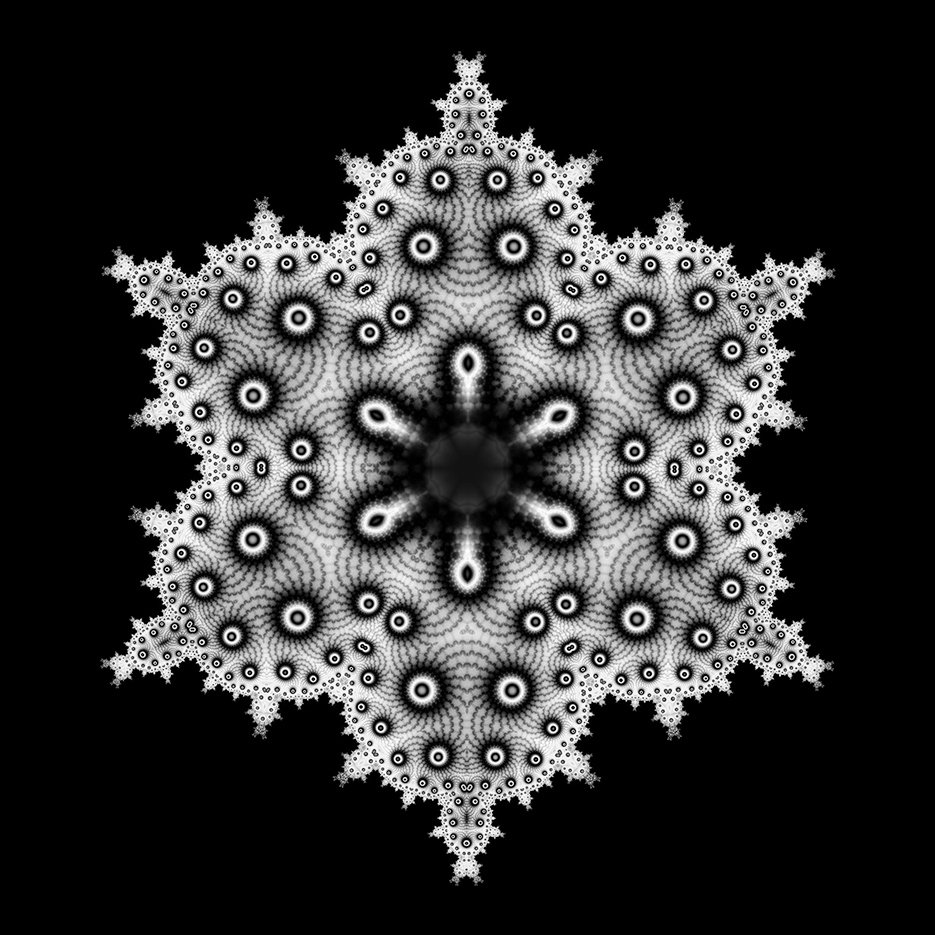
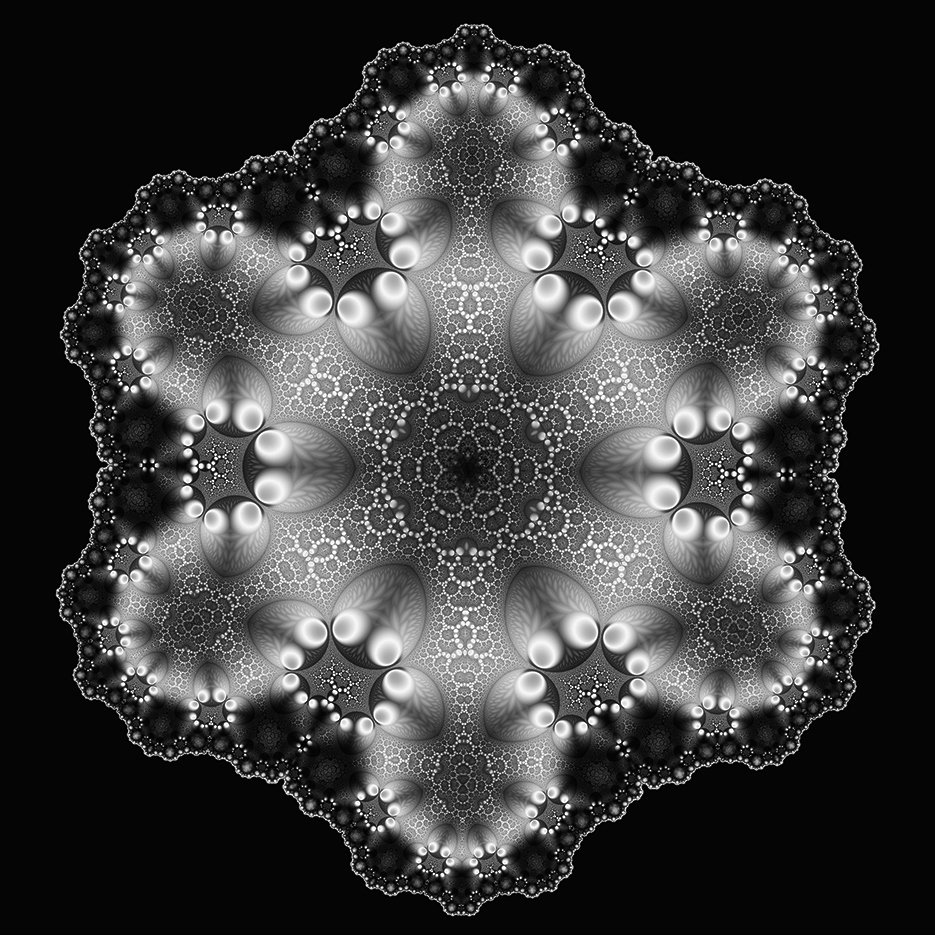
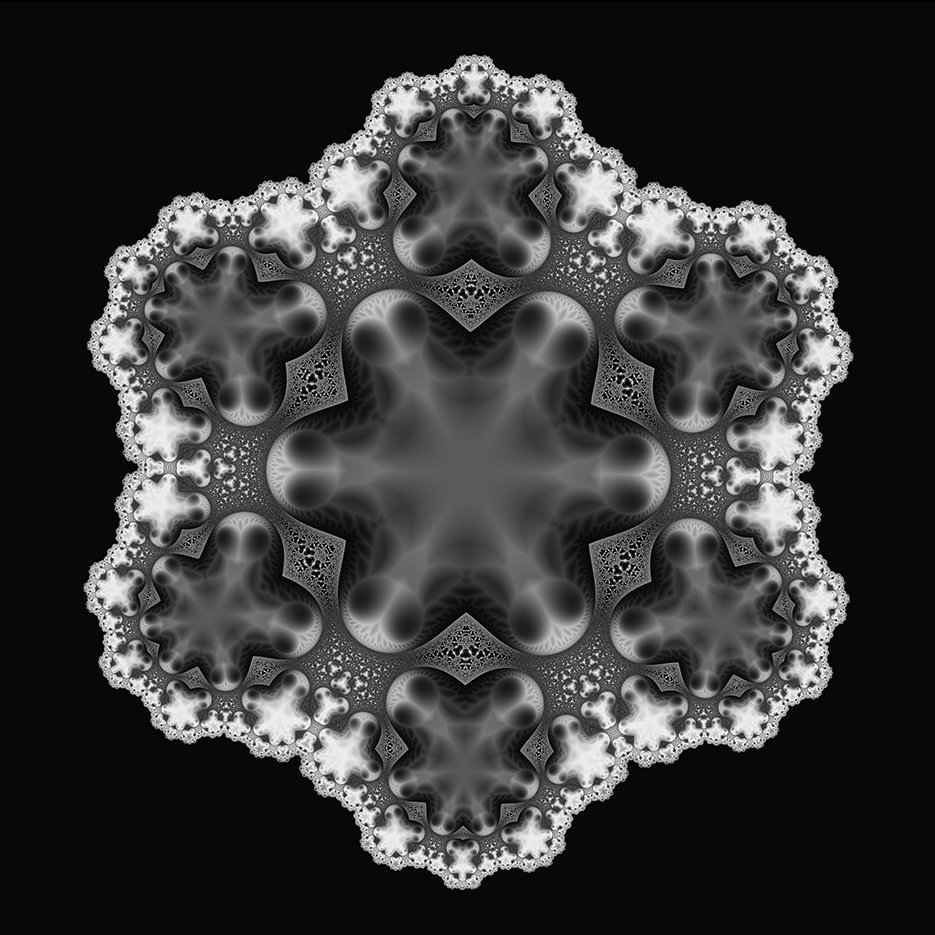
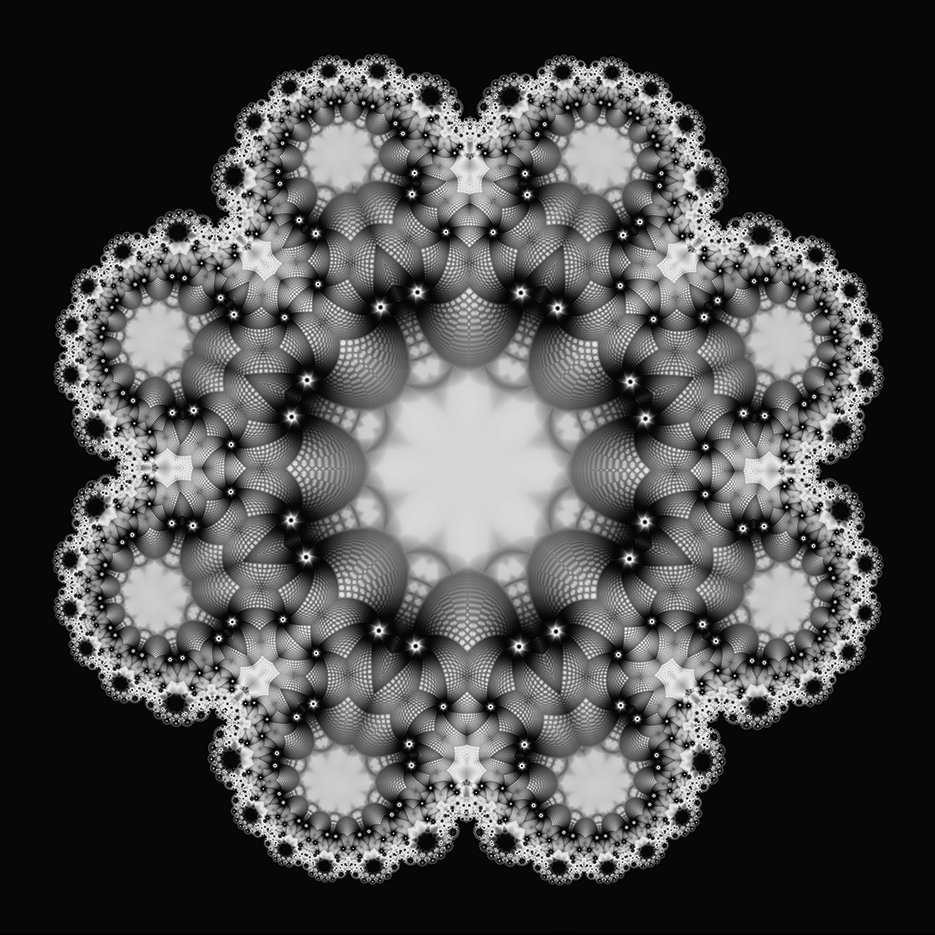
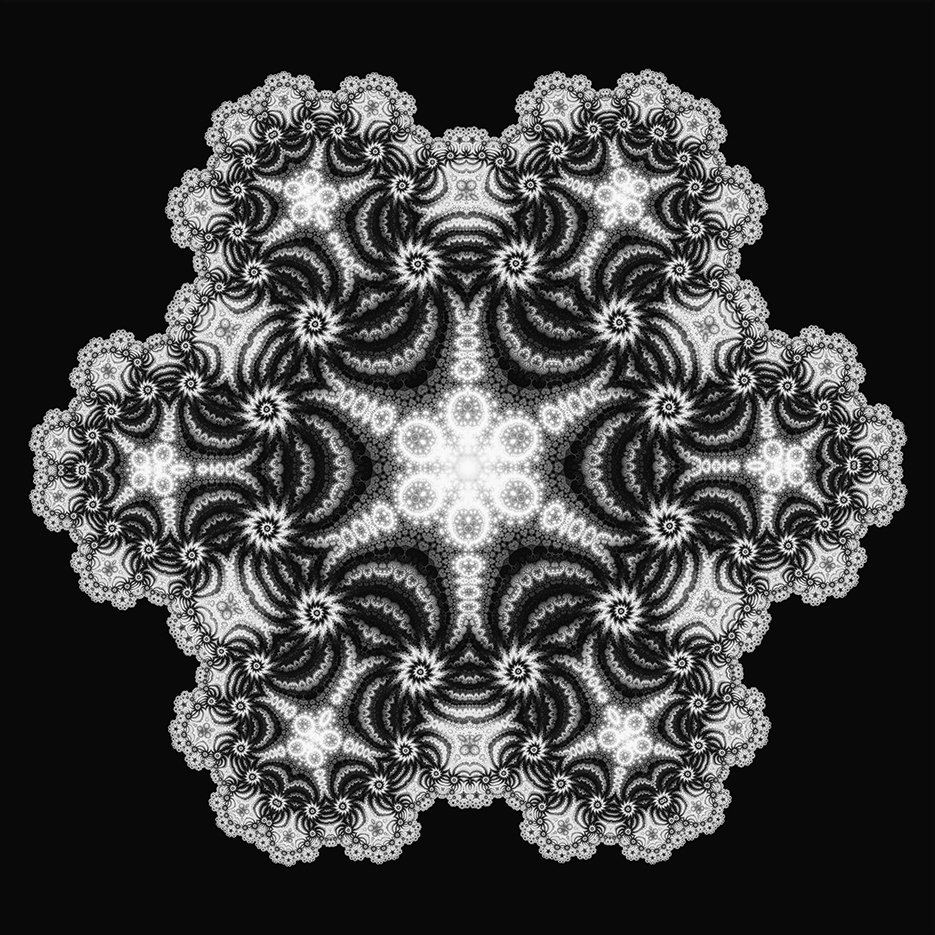
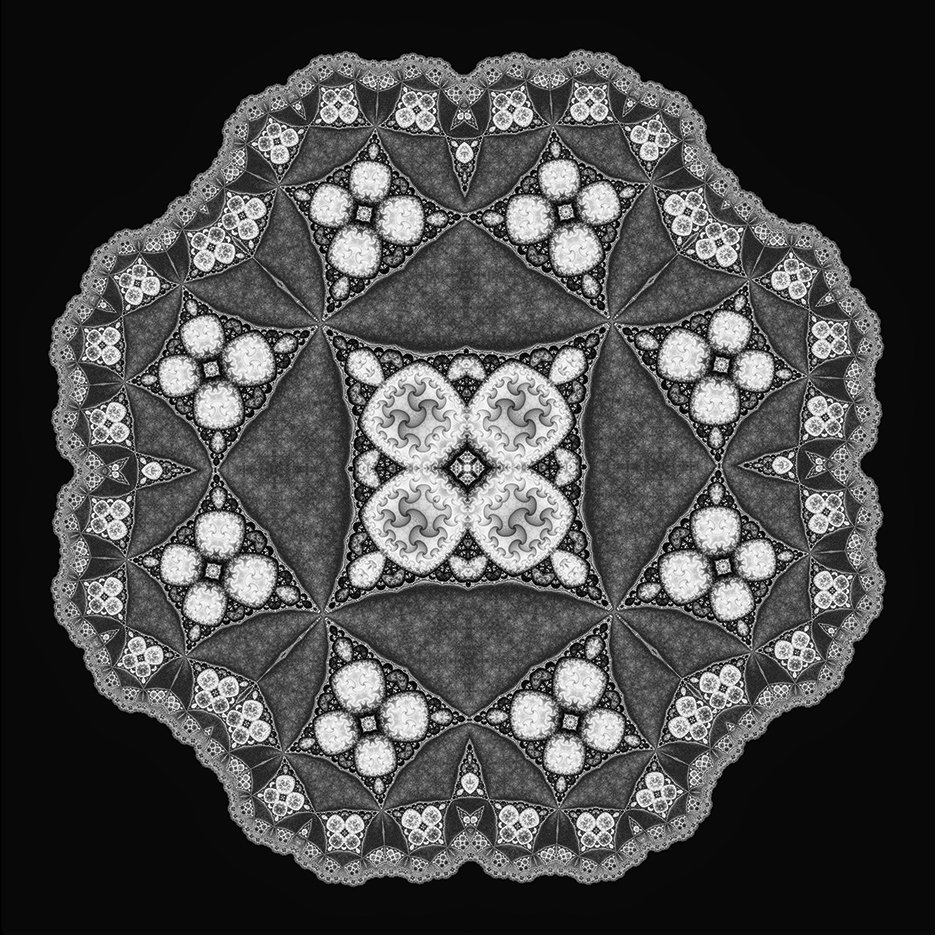
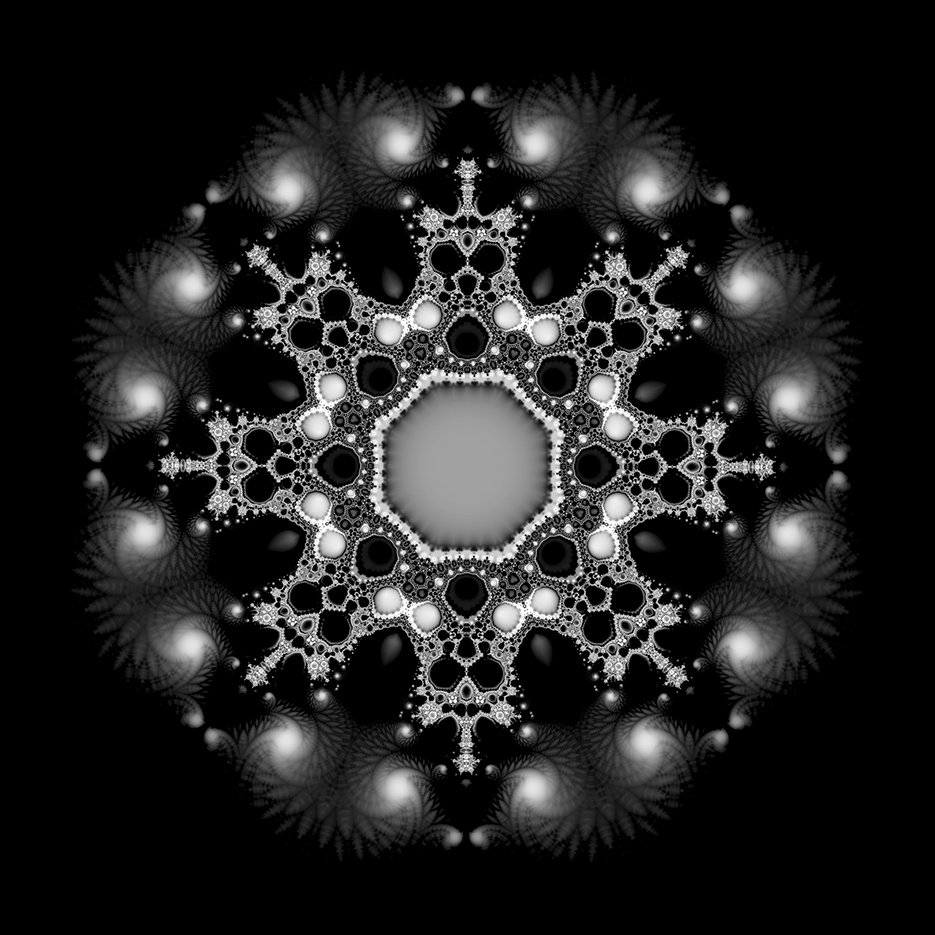
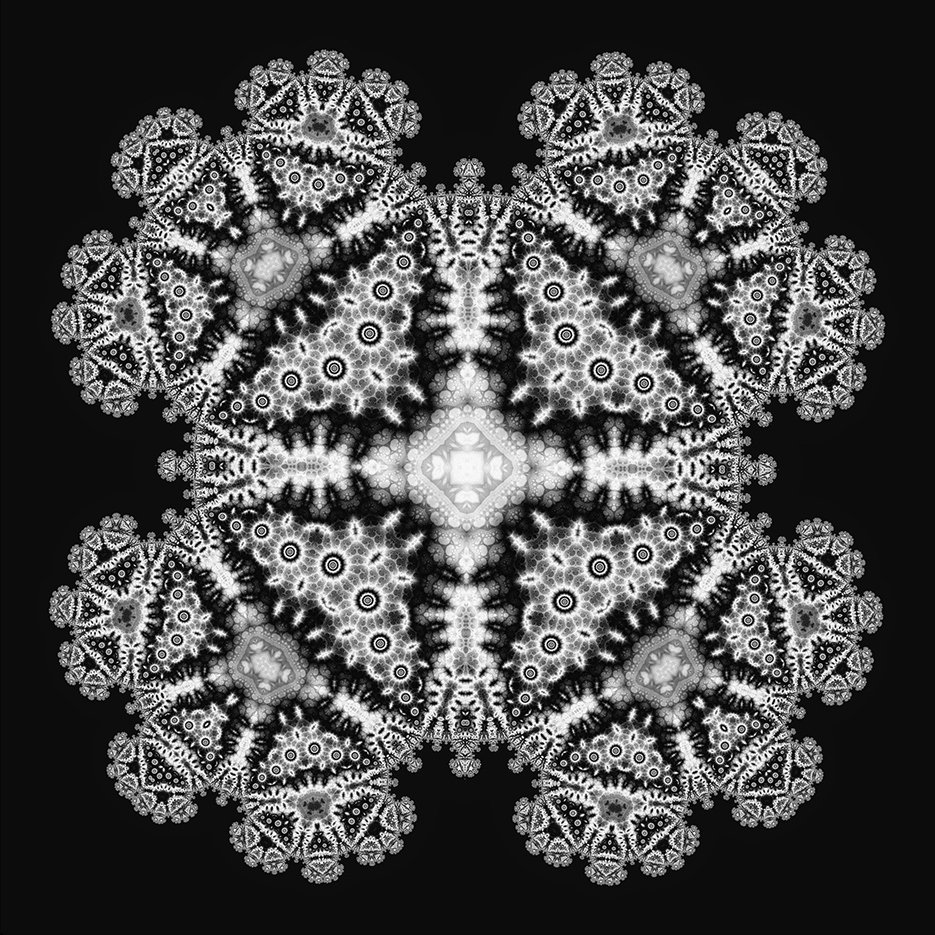
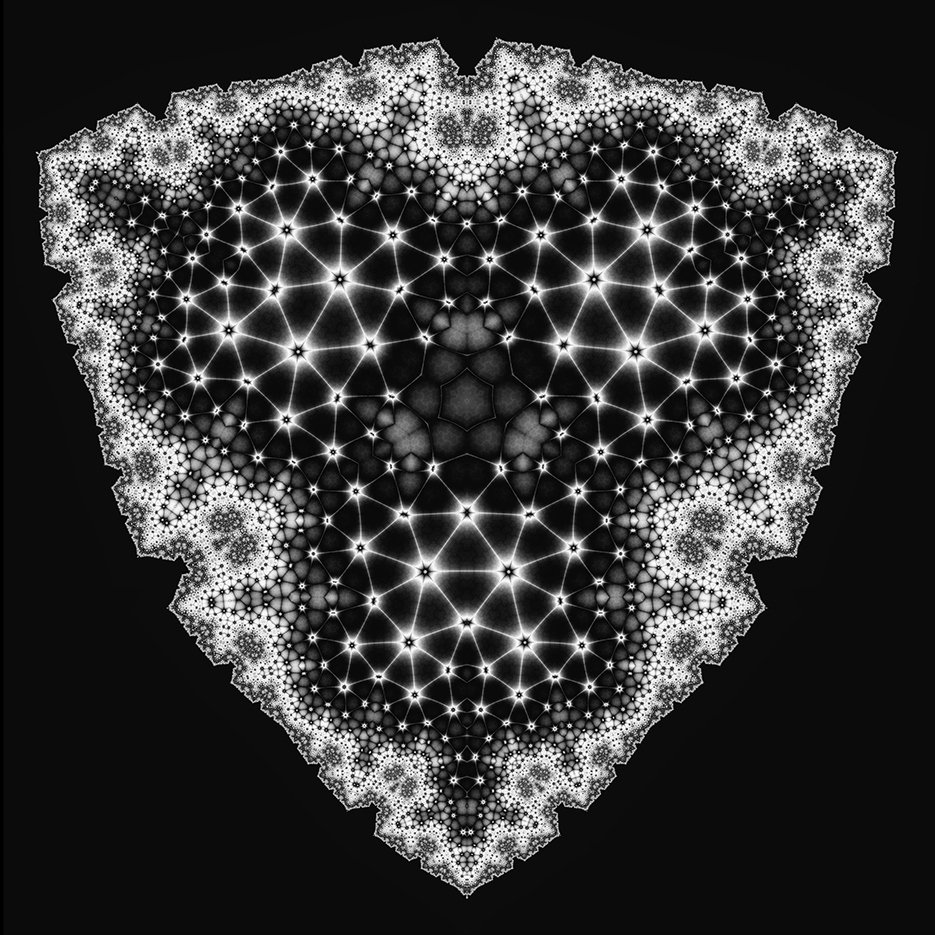
Or maybe just a patchwork of patterns...
Related posts
- Introducing Frax
- The project I've been working on for the last two years...
- L'Eclaireur
- A series of looping animations from an installation at L'Eclaireur in Paris.
- Music Box
- A journey into a Fabergé inspired world.
- Fractal Lab
- A WebGL fractal explorer
- More blog posts
It looks like a Mandelbrot Set.
Those are wonderful structures !
These invoke the feeling of being 2D crosscut sections
of utterly complex 3D objects...
...but actually they are indications
that you Tom are a 3D shadow of an utterly complex 4D being ;)
It has been great fun to get to know you
well, a little crosscut of you, at least...
MC Escher is smiling at this from the impossible heavens
Cheers, Kai Krause
Beautiful work Tom!
Beautiful and stunning!!!
Lovely
wow, simply beautiful work!
Beautiful!
Is it a julia set and foldings mix?
They are all julia-style sets at various powers with an abs() term to effectively fold:
z' = abs(a * z^p) * b + c
where p is real but a, b, c are complex.
I'm also using a standard accumulative exponential index for colouring:
idx += exp(-1/abs(d))
where d is the difference between length of the current and previous value of z.
Thank you.
Thanks, Tom! You just gave me the perfect material for my FMX13 talk "The fallacy of the snowflake".
Cheers!
F.
Realy enthralling and captivating images. I spent a good half an hour looking through them individually and found them totally encapsulating.
Thanks
Stunning images.
I was just thinking about a mental puzzle
unit area vs. circumference (perimeter length)
of various regular polygons.
Show these polygons of unit area,
and show the circumference:
(or sum of length of sides)
Circle=3.5
Pentagon=3.8
Square=4
Equilateral triangle=4.5
Then ask the student:
Is it possible to construct a polygon
(closed arc)
with a unit area=1
with a perimeter length > 4.5?
And the answer is of course YES.
I believe it is possible for
one to construct a closed arc
of unit area with a fractal boundary
of any arbitrary length from 4.5 to infinity.
Thanks for the pretty pictures!